Fx Graph Examples
Take the graph for positive values of x, and flip it over the y-axis to get the graph for negative values of x.;.
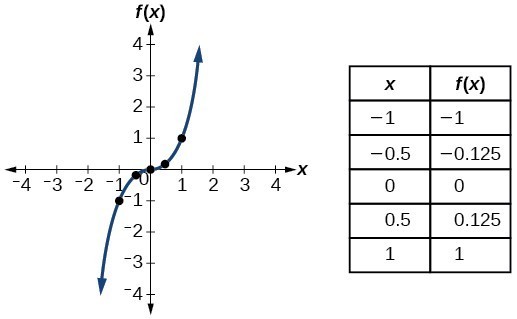
Fx graph examples. The following table shows several values for x and the function f evaluated at those numbers. Linear functions have the form f(x) = ax + b, where a and b are constants. Such a function is referred to as a constant function.
Find the intercepts. Multiple Choice The function on the left is. Also note that the graph shoots upward rapidly as x increases.
If f is decreasing on an interval, then f ' < 0 (below the x-axis) in that interval. Ignore the left hand side part of the graph In this video I show you how to draw graphs of the form y=f(|x|) using the modulus function and give you three graphs to try. A) Graph x = –3.
R → R We find various values of f(x) by using different values of x Domain is a real number Range is a Real number The graph of the function is. Well instead of flipping the y values, you want to flip the x values. For example, if we begin by graphing the parent function f (x) = 2 x, f (x) = 2 x, we can then graph the stretch, using a = 3, a = 3, to get g (x) = 3 (2) x g (x) = 3 (2) x as shown on the left in Figure 8, and the compression, using a = 1 3, a = 1 3, to get h (x) = 1 3 (2) x h (x) = 1 3 (2) x as shown on the right in Figure 8.
F(x) = x 2. Graph the logarithmic function f(x) = log 2 x and state range and domain of the function. Desmos offers best-in-class calculators, digital math activities, and curriculum to help every student love math and love learning math.
The graph of f (blue) and f '' (red) are shown below. However, the set of all points (x,y) (x, y) satisfying y =f (x) y = f (x) is a curve. From the graph of f ' (x), draw a graph of f(x).
The graph of any quadratic function f (x) = a x 2 + b x + c, where a, b, and c are real numbers and a ≠ 0, is called a parabola.;. Let f (x) = 2 x + 2 and compute f (x) as x takes values closer to 1. To understand the behavior of this function, in terms of a graph, let's construct a table of values.
Visual Effect Graph empowers you to author next-generation visual effects through its node-based behaviors and GPU-based compute power. C > 0 moves it up;. The point x=a determines an absolute maximum for function f if it corresponds to the largest y-value in the range of f.
But drawing the graph with a planar representation shows that in fact there are only 4 faces. For example, f (3) = 9, and f (–3) = 9. If f is increasing on an interval, then f ' > 0 (above the x-axis) in that interval.
To move the line down, we use a negative value for C. A function f(x) is called odd if its value at -x is the negative of its value at x. Graphs of f (x), f (x) + 2, and f (x) - 2.
Y=mx+c Where m is the slope of the line and c in the y-intercept. Example — Estimating the Graph of a Derivative. A function f(x) is called even if its value at x is the same as its value at -x.That is, if f(x) = f(-x).An even function has a graph that is symmetric about the y-axis:.
Sketch a graph of a counter example to the following statement. Transformations involving $\,y\,$ work the way you would expect them to work—they are intuitive. Plot the intercepts, label each point, and draw a straight line through these points.
Since the initial preview release at Unite LA 18, we’ve also been publishing various sample VFXs to our GitHub repository. Shifting to the right works the same way;. Example 8 Graph of Graph of.
In latexf\left(x\right)=mx+b/latex, the b acts as the vertical shift, moving the graph up and down without affecting the slope of the line. Where -3x+6 =0 -> x=2 Thus, to draw the graph of F(x) simply. Here is the thought process you should use when you are given the graph of $\,y=f(x)\,$.
This is because the function must compensate. F(|x|) reflects the graph to the right of the y-axis in the y-axis. Graph of a hyperbolic paraboloid.
The simplest case, apart from the trivial case of a constant function, is when y is a linear function of x, meaning that the graph of y is a line. The graph of f (x) in this example is the graph of y = x 2 - 3. In other words, f(x) = ax + b is increasing on (− ∞, ∞).
B) Decide whether the graph represents a function. While adding to the input increases the function in the y direction, adding to the input decreases the function in the x direction. If f(x) has a critical number at x=a and f"(a) is not positive, then a is the location of a local maximum of f(x).
Formally, a function f from a set X to a set Y is defined by a set G of ordered pairs (x, y) such that x ∈ X, y ∈ Y, and every element of X is the first component of exactly one ordered pair in G. In Figure 1.1.1, we see examples of linear functions when a is positive, negative, and zero. Find the properties of the given parabola.
For the function f(x) below, draw a graph of f ' (x).Don't worry too much about whether f ' is straight or curvy—focus on getting it to cross the x axis in the right places. Tap for more steps. Euler's Formula for Planar Graphs.
More information about applet. Use the form , to find the values of , , and. If f turns around smoothly at a point x = a, then f '(a) = 0 (intersecting the x-axis).
The graph of y = c, or f (x) = c, a horizontal line, is the graph of a function. So you replace the x with minus x and that will reflect the graph across the y axis. Intuitively, a function is a process that associates each element of a set X, to a single element of a set Y.
Notice in Figure 5 that adding a value of b to the equation of latexf\left(x\right)=x/latex shifts the graph of f a total of b units up if b is positive and |b| units down if b is negative. (iii) The graph of y = f −1 (x) is the reflection of the graph of f in y = x. The above even function is equivalent to:.
When graphing a parabola always find the vertex and the y-intercept.If the x-intercepts exist, find those as well.Also, be sure to find ordered pair solutions on either side of the line of symmetry, x = − b 2 a. Which graph on the right is ?. We first consider values of x approaching 1 from the left (x < 1).
F(x) = -3x+6 Hence, y =F(x), m=-3 and c=+6 Therefore the graph of F(x) is a straight line with slope of -3 and y-intercept (0,6) Also note that the x-intercept occurs where F(x)=0 I.e. See below The equation of a straight line in Slope/Intercept is:. C < 0 moves it down.
F (x + 3) = (x + 3) 2 is f (x) shifted three units to the left. Find the y-intercept, let x = 0 then substitute 0 for x in the equation and solve for y Step 3:. The point x=a determines a relative minimum for function f if f is continuous at x=a, and the first derivative f' is negative (-) for x<a and positive (+) for x>a.
In fact, it intersects the x-axis at the right place right over there. Here are some simple things we can do to move or scale it on the graph:. Tap for more steps.
Some useful reflections of y = f(x) are (i) The graph y = −f(x) is the reflection of the graph of f about the x-axis. In this graph, f (x) has been moved over three units to the left:. An odd function has the property f(−x) = −f(x).
This graph is positive when the slope of the tangent line here is positive. The graph of x = a, a vertical line, is not the. Horizontal Shifts If c is a positive real number, then the graph of f (x + c) is the graph of y = f (x) shifted to the left c units.
This means f will be decreasing for a bit, and will then turn around and increase:. We now consider x approaching 1 from the right (x > 1). Thus, the graph of $\,y=f(x)+3\,$ is the same as the graph of $\,y=f(x)\,$, shifted UP three units.
This isn't really a functions-operations question, but something like this often arises in the functions-operations context. G(x) = x 2 + C. Linear, quadratic, square root, absolute value and reciprocal functions, transform parent functions, parent functions with equations, graphs, domain, range and asymptotes, graphs of basic functions that you should know for PreCalculus with video lessons, examples and step-by-step solutions.
When you find (f o g)(x), there are two things that must be satisfied:. So let's consider an example y=2 to the negative x. Examples in the video:.
The graph of the function $f(x,y)=x^2-y^2$. F(x) = x3, x ∈ R Also, f:. An inverse function goes the other way!.
It can easily be seen that whenever f '' is negative (its graph is below the x-axis), the graph of f is concave down and whenever f '' is positive (its graph is above the x-axis) the graph of f is concave up. And this graph is negative when the slope of the tangent line here is negative. Learn how we analyze a limit graphically and see cases where a limit doesn't exist.
For example, the black dots on the graph in the graph below tell us that f (0) = 2 f (0) = 2 and f (6) = 1 f (6) = 1. = (+) − (). Solve the equation $$\lfloor 2x + 5\rfloor = 9$$.
Every point of f has a corresponding image in f'. Complete the square for. So, for example, $$\lfloor x\rfloor = 8$$ if and only if $$8 \leq x.
For starters, we released an introduction blog post that summarizes the philosophy of the editor. It is easy to generate points on the graph. F (x + b) gives f (x) shifted b units to the left.
Given f (x) = 3x 2 – x + 4, find the simplified form of the following expression, and evaluate at h = 0:. $$ \lim\limits_{x\to-\infty} f(x) \approx 8 $$ When Limits at Infinity Don't Exist In order for a limit at infinity to exist, the function must approach a particular finite value. The simplest example of this is f (x) = x2 because f (x)=f (-x) for all x.
The graph of inverse function of any function is the reflection of the graph of the function about the line y = x. Visually speaking, the graph is a mirror image about the y- axis, as shown here. F ' is negative, then zero, then positive:.
Note that if a > 0, the graph of the line rises as x increases. Worked example matching a function, its first derivative and its second derivative to the appropriate graph. Graph f(x)=|x| Find the absolute value vertex.
So the inverse of:. Estimating limit values from graphs. This is because of the doubling behavior of the exponential.
Basically, the opposite input yields the same output. How to find intercepts and use intercepts to graph linear equation?. Google Classroom Facebook Twitter.
There is a connection between the number of vertices (\(v\)), the number of edges (\(e\)) and the number of faces (\(f\)) in any connected planar graph. This is a reflection of what parent function?. Let $f(x,y,z) = x^2+y^2+z^2$.
So, the graph of the logarithmic function y = log 3 ( x ) which is the inverse of the function y = 3 x is the reflection of the above graph about the line y = x. Given the graph of the derivative, sketch a possible graph for the function. B) Decide whether the graph represents a function.
Exponential Decay In the form y = ab x, if b is a number between 0 and 1, the function represents exponential decay. We can move it up or down by adding a constant to the y-value:. Multiple Choice The function on the left is.
Obviously, a logarithmic function must have the domain and range of (0,infinity) and (−infinity, infinity) Since the base of the function f(x) = log 2 x is greater than 1, we will increase our curve from left to right a shown below. Find the intercepts of 3x + y = 6 2. Y=-f of x flips the graph across the x axis.
In this case, y = f(x) = mx + b, for real numbers m and b, and the slope m is given by = =, where the symbol Δ is an abbreviation for "change in", and the combinations and refer to corresponding changes, i.e.:. In this case, the vertex for is. F (x – b) is f (x) shifted b units to.
This is always true:. An example of an even function is f(x) = x 4 − 29x 2 + 100. This relationship is called Euler's formula.
For example, (1, 2) is on the graph of f (x), (1, 4) is on the graph of f (x) + 2, and (1, 0) is on the graph of f (x) - 2. Let us start with a function, in this case it is f(x) = x 2, but it could be anything:. If c is a positive real number, then the graph of f (x – c) is the graph of y = f (x) shifted to the right c units.
To shift a function left, add inside the function's argument:. The Inverse Function goes the other way:. The basic shape of an exponential decay function is shown below in the example of f(x) = 2 −x.
(ii) The graph y = f(−x) is the reflection of the graph of f about the y-axis. And at least over this interval, it seems it's positive from here to here. The best way to start reasoning about limits is using graphs.
In both cases as x approaches 1, f (x) approaches 4. Graph x - y = 5 3. Choose a value for the first coordinate, then evaluate f at that number to find the second coordinate.
A) Graph y = 4. When you combine the two domains to see what they have in common, you find the intersection of everything and nothing is. Estimating limit values from graphs.
Example 14 Draw the graph of the function f :R → R defined by f (x) = x3, x ∈ R. Use the leading coefficient, a, to. X must be in the domain of g, which means x is a real number (pretty easy to do) g(x) must be in the domain of f, which means that 1-x 2 ^2 ≥ 4 (when you try to solve this, you get the empty set);.
Rewrite the equation in vertex form. Tap for more steps. Graph on the right.
Let us start with an example:. Sketch the following latexy. But how do you reflect it across the y axis?.
We just don't know exactly where the graph of f(x) will be in relation to the y-axis.We can figure out the general shape of f, but f we could take the graph of f that we just made and shift it up or down along the y. F(x) = (x + 5)(x + 2)(x − 2)(x − 5) Note if we reflect the graph in the y-axis, we get the same graph (or we could say it "maps onto" itself).

Transformations Mrs F X
Function Definition Types Examples Facts Britannica

Ppt Graph Transformations Powerpoint Presentation Free Download Id
Fx Graph Examples のギャラリー

Using Transformations To Graph Functions

Common Functions Reference

Derivatives Of Inverse Functions
Q Tbn 3aand9gcrofuq5rtdmiqeg8p0hgzpnmolssoksapaqdongvfnhrzggkapg Usqp Cau

Derivatives In Curve Sketching

Combining Transformations Read Algebra Ck 12 Foundation
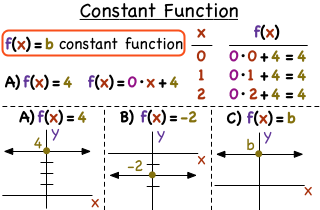
What Is A Constant Function Printable Summary Virtual Nerd

Calculus Revision Maths First Institute Of Fundamental Sciences Massey University
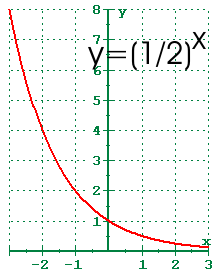
4 1 Exponential Functions And Their Graphs
Finding Limits Graphically
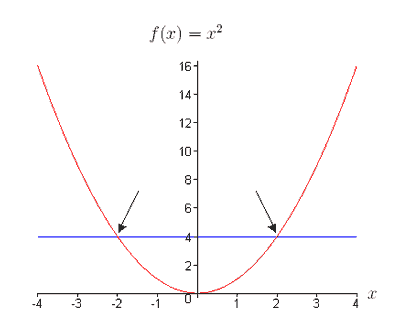
What Is The Graph Of F X X 2 Example
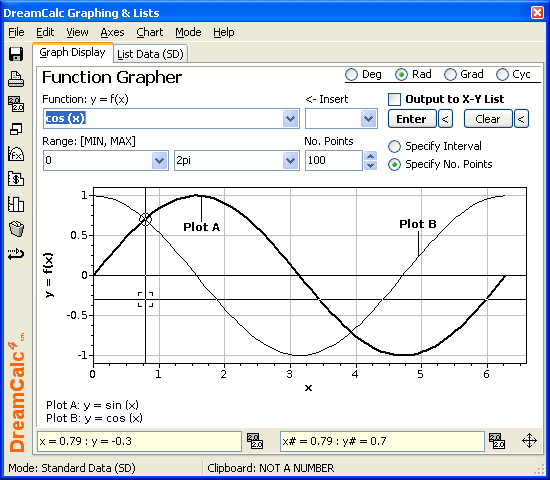
Graphing Calculator Graphing Examples
Q Tbn 3aand9gcsra5g1kcbxvfmlnm3m0pljc4lmqknjfqj9sw19mefkaoos2kbv Usqp Cau
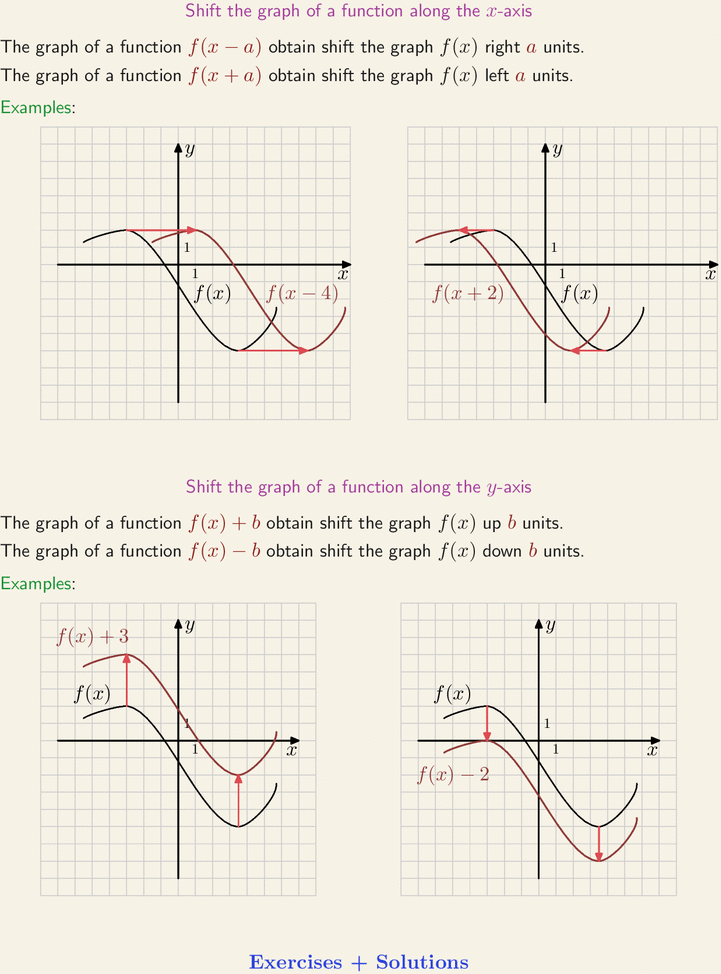
Shift The Graph Of A Function Along X And Y Axis

Even And Odd Functions

Graph Of F X 2x 4 Linear Function Graphing Math Boards

Reading The Derivative S Graph Teaching Calculus
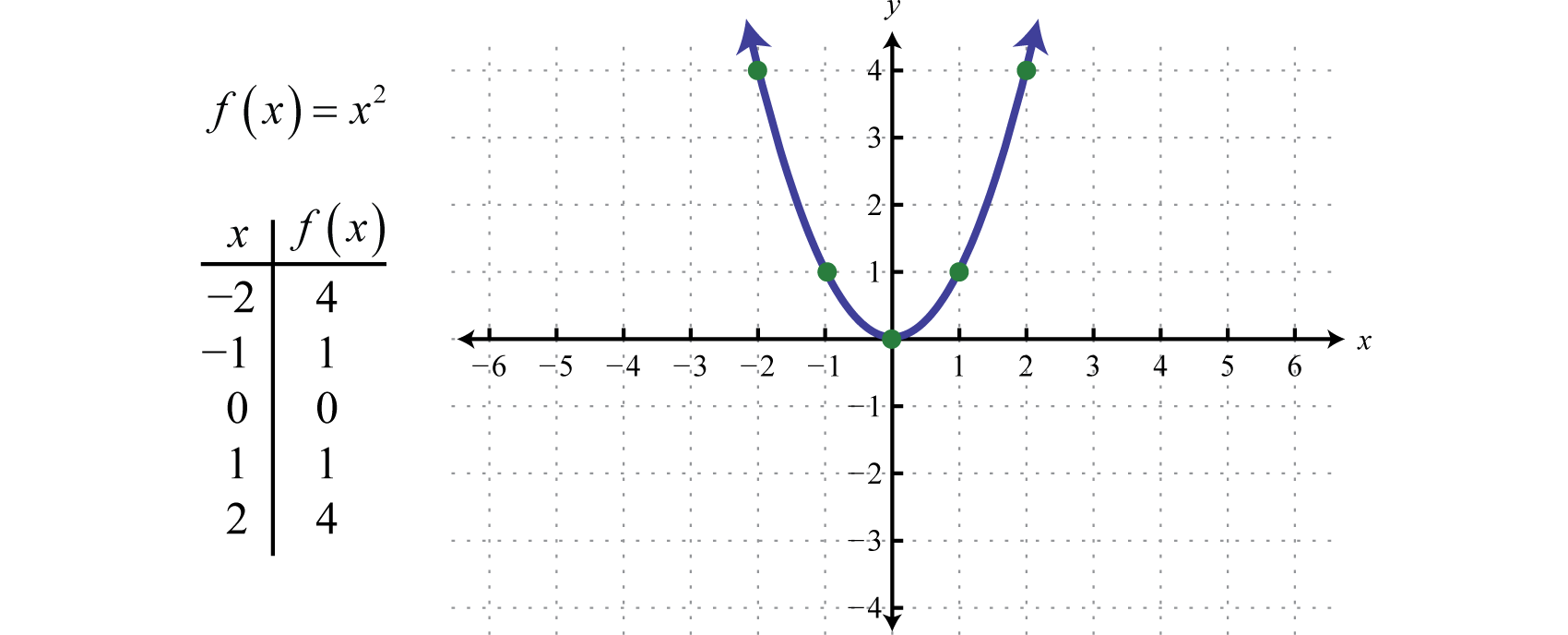
Quadratic Functions And Their Graphs
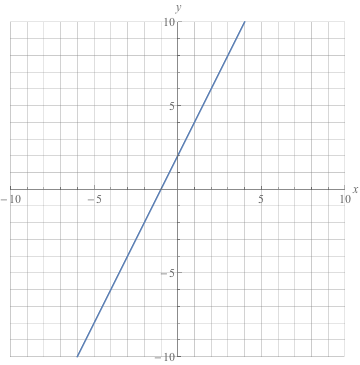
Graphs Of Em F Em Em X Em And Em F Em Em X Em Examples

Graphs Of Functions
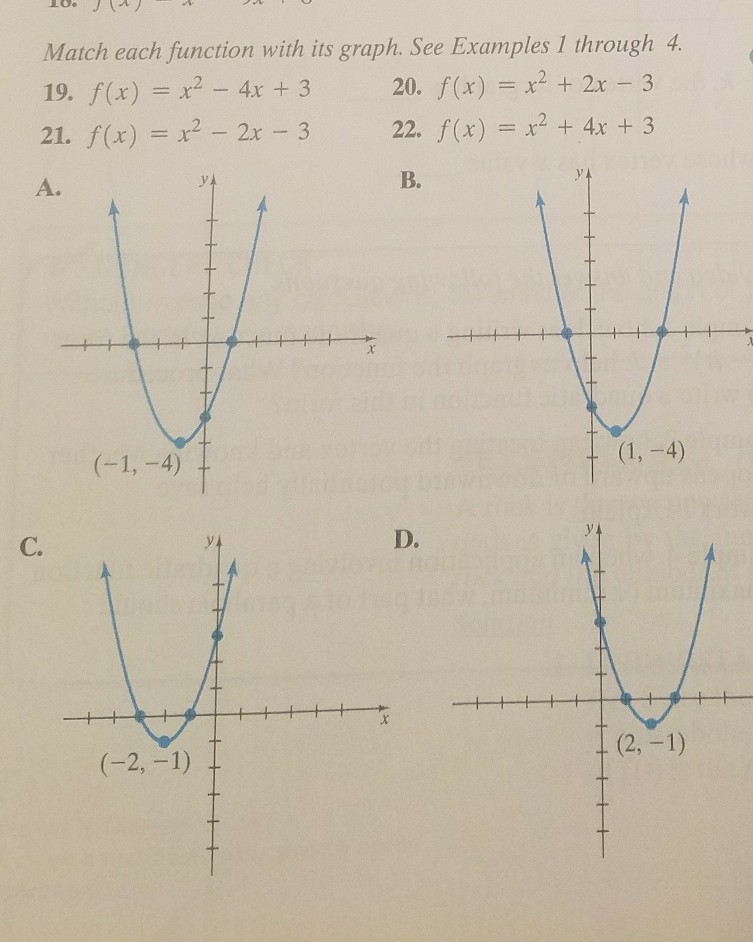
Solved Match Each Function With Is Graph See Examples I Chegg Com
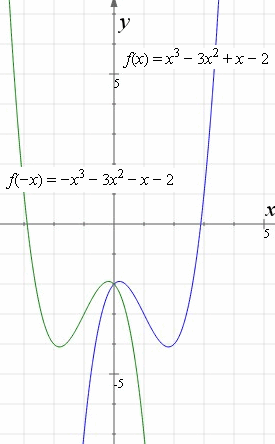
How To Reflect A Graph Through The X Axis Y Axis Or Origin

Example Of Area Bounded By Graph Y F X And Limits X A X B And Download Scientific Diagram
Biomath Functions
Reflect Function About Y Axis F X Expii
Graphing Shifted Functions Video Khan Academy
How To Reflect A Graph Through The X Axis Y Axis Or Origin
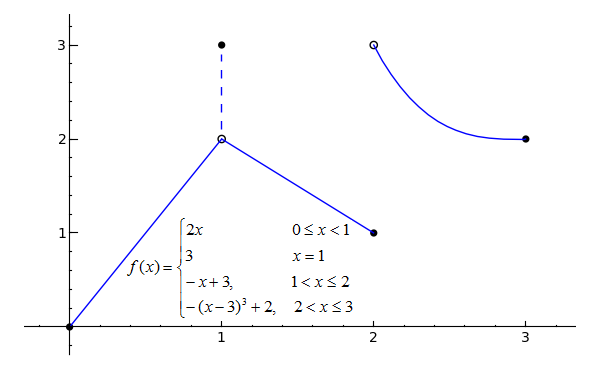
Sage Calculus Tutorial Continuity
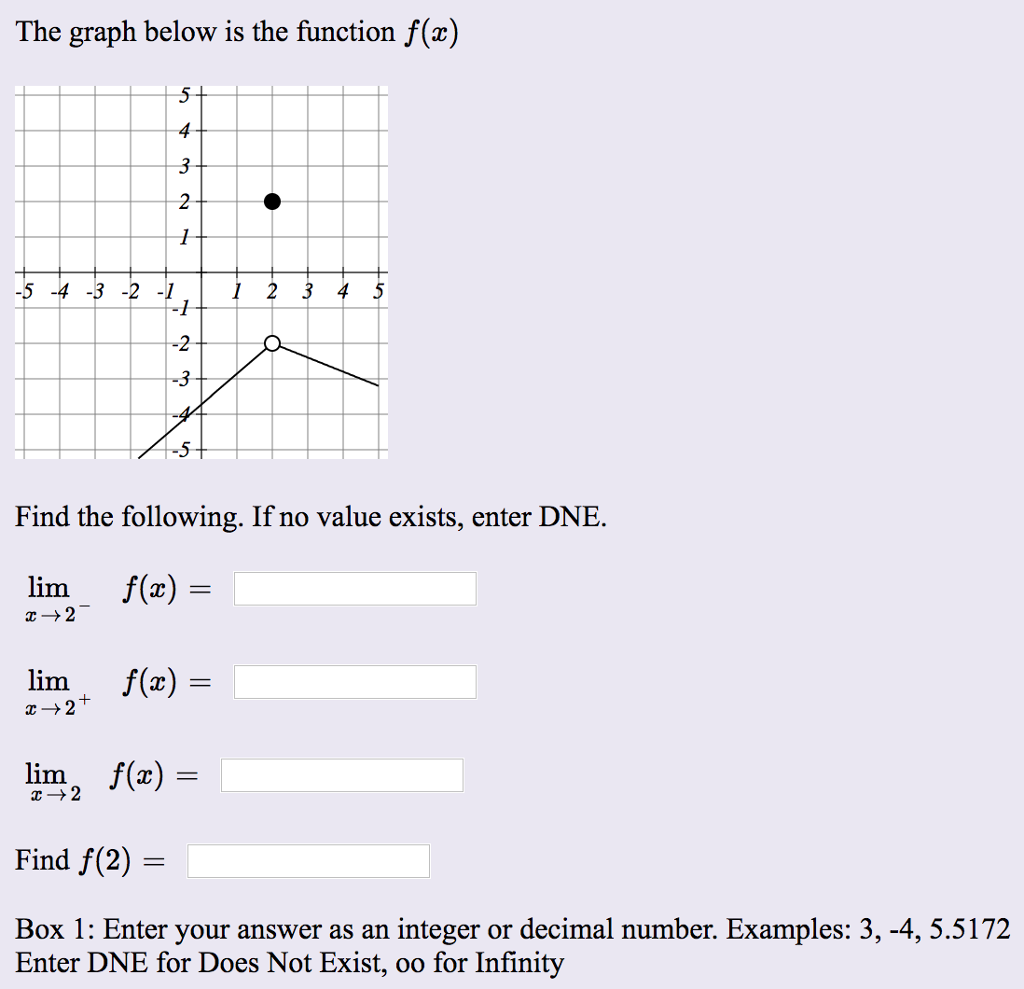
Solved The Graph Below Is The Function F X Find The Foll Chegg Com
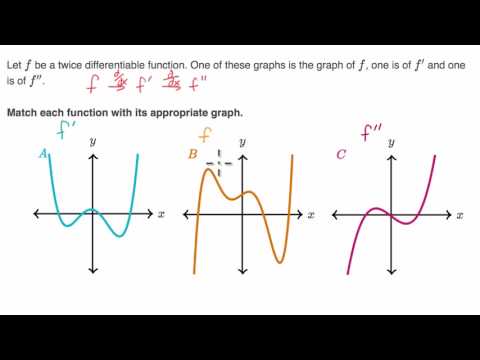
Identifying F F And F Based On Graphs Youtube
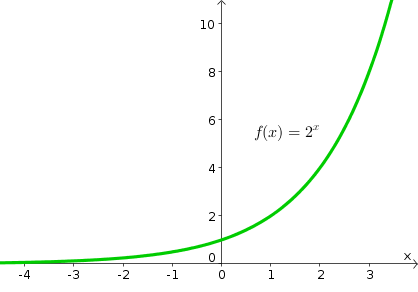
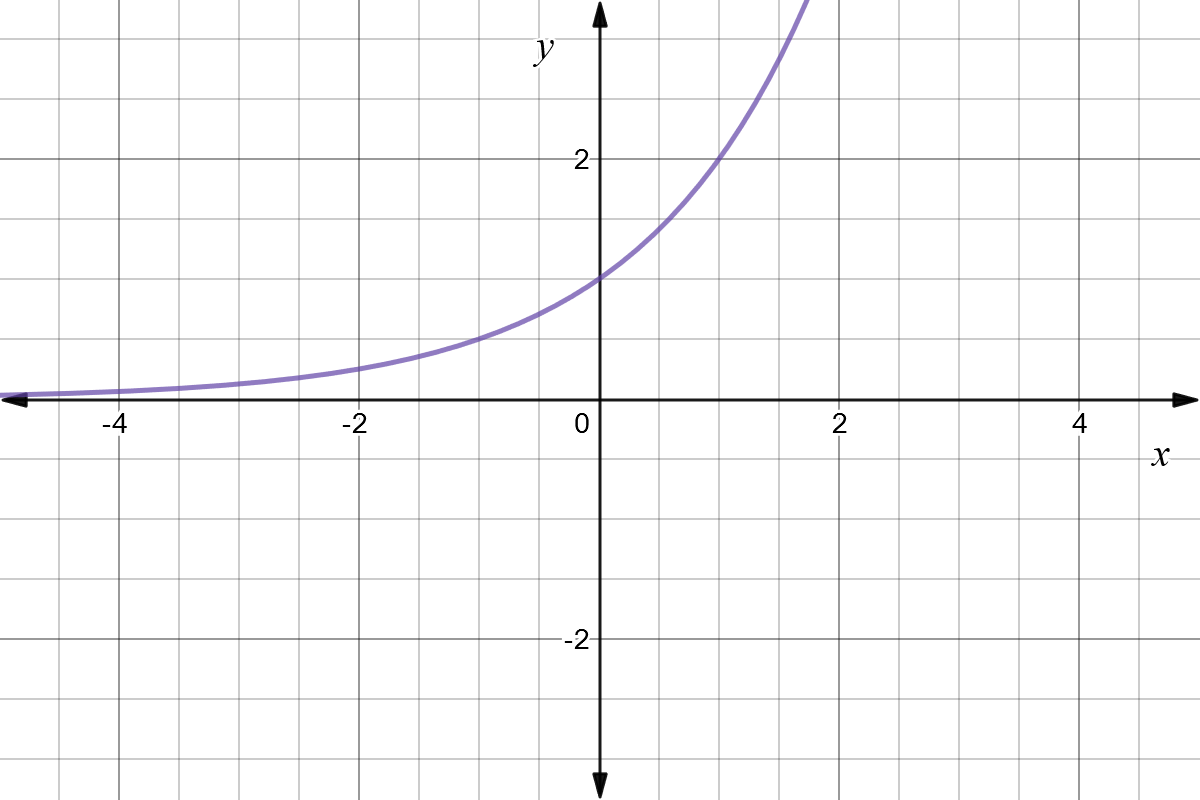
The Exponential Function Math Insight
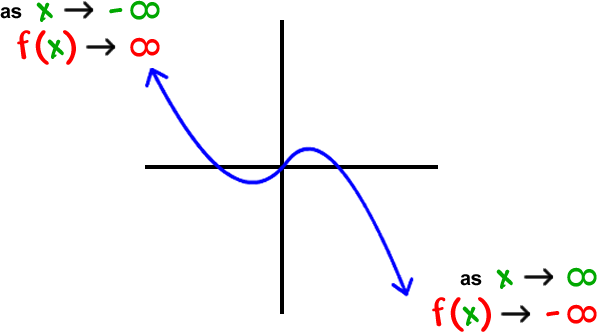
Estimating Limit Values With Graphs

Graphing Square Root Functions
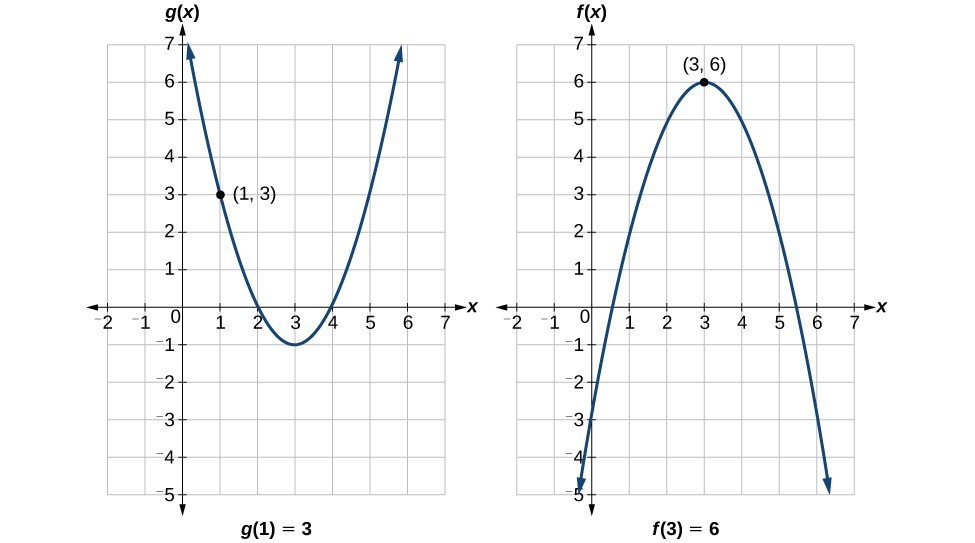
Evaluate Composite Functions College Algebra
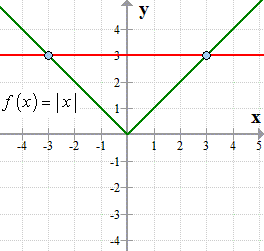
Inverse Of Absolute Value Function Chilimath

Graphs Of Exponential Functions Brilliant Math Science Wiki
Q Tbn 3aand9gcsmm4ktmxrxm10kmsg3wqo5rzire Ogwbm0v2tajixhbesgniod Usqp Cau
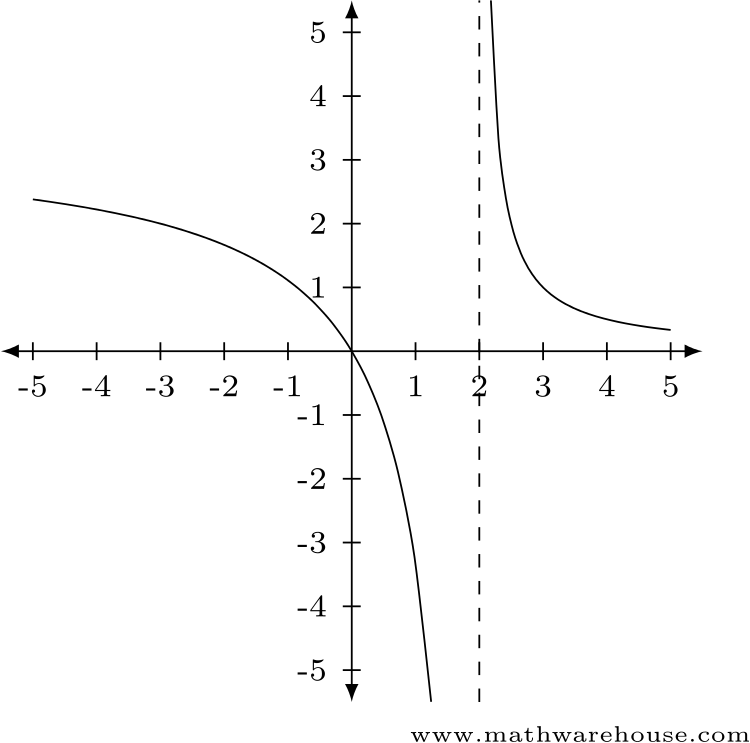
Rational Functions Limits 1
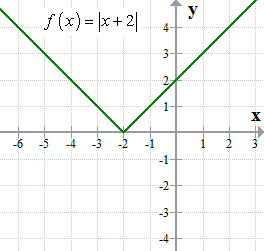
Inverse Of Absolute Value Function Chilimath
Curve Sketching
Www Weber Edu Wsuimages Mtc Handouts Transformations Generic Pdf
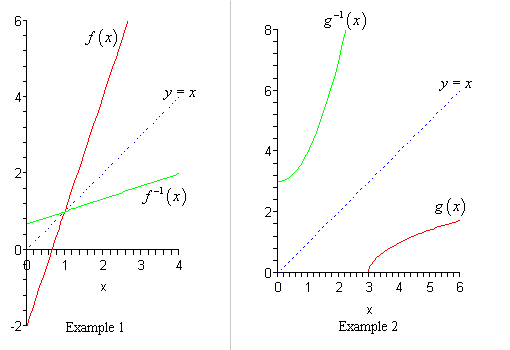
Algebra Inverse Functions
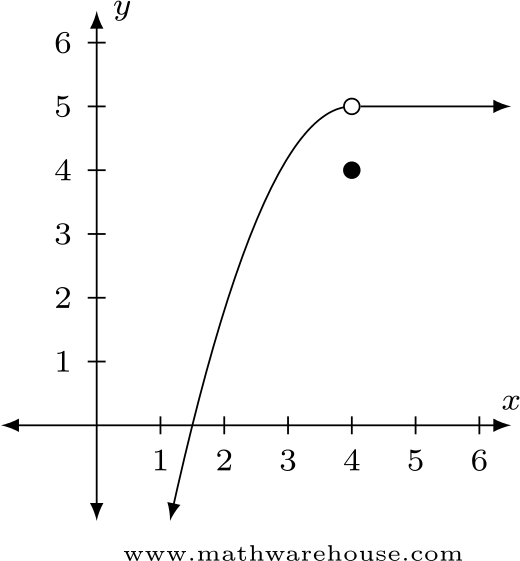
Estimating Limit Values With Graphs
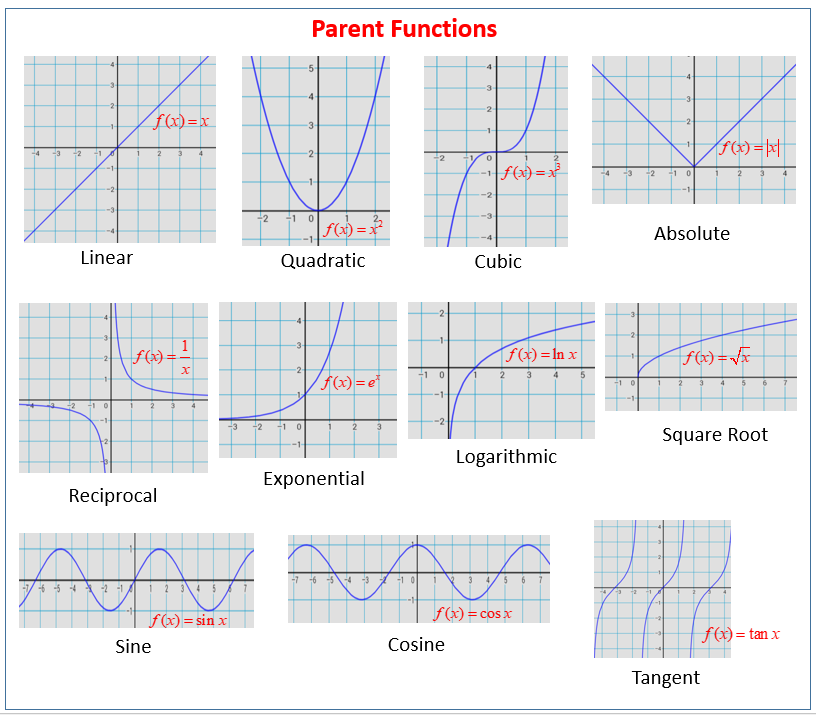
Parent Functions And Their Graphs Video Lessons Examples And Solutions
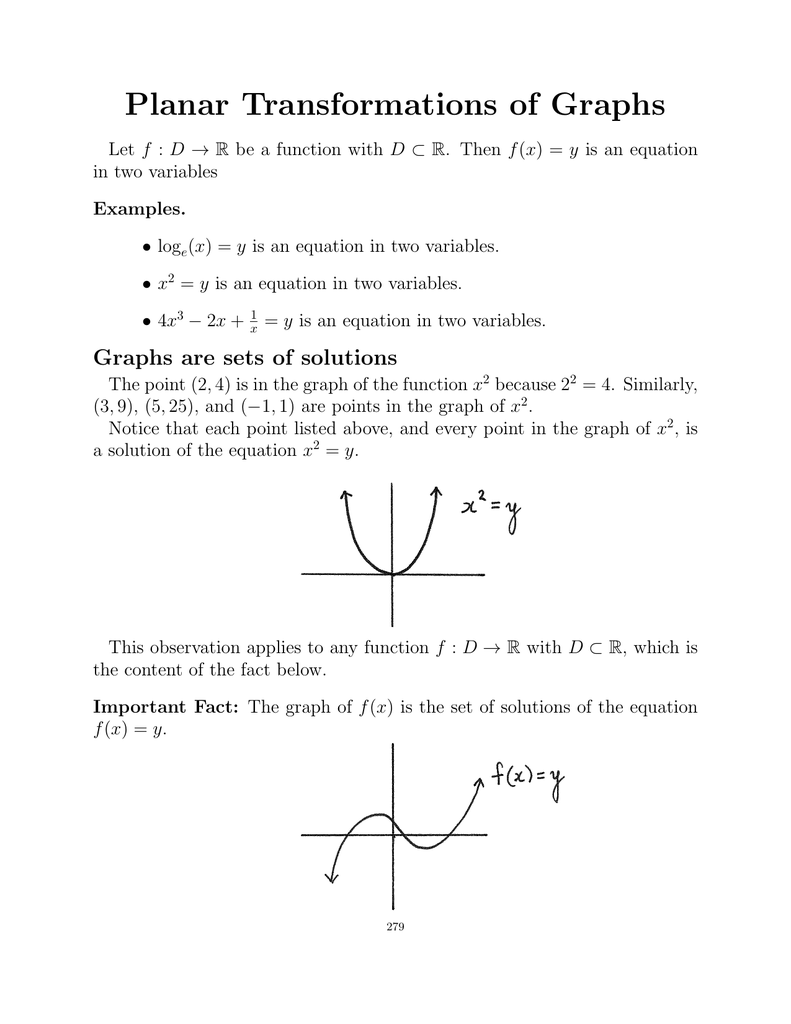
Planar Transformations Of Graphs F X F
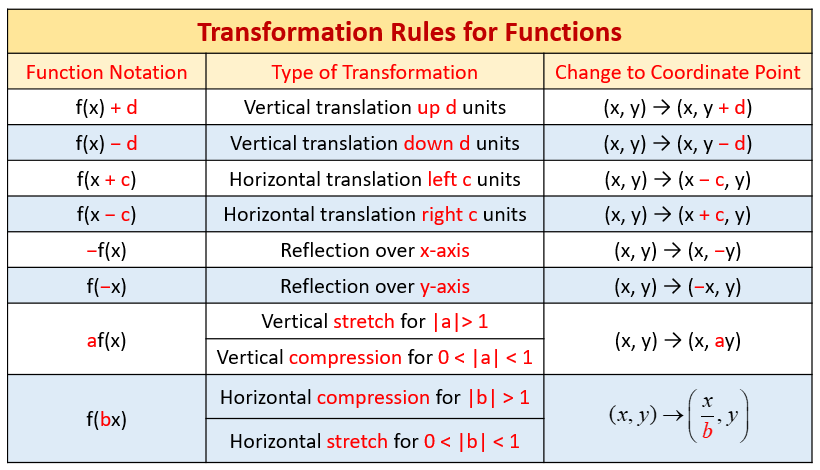
Parent Functions And Their Graphs Video Lessons Examples And Solutions

Comparing Function Transformations Study Com
Q Tbn 3aand9gcsra5g1kcbxvfmlnm3m0pljc4lmqknjfqj9sw19mefkaoos2kbv Usqp Cau
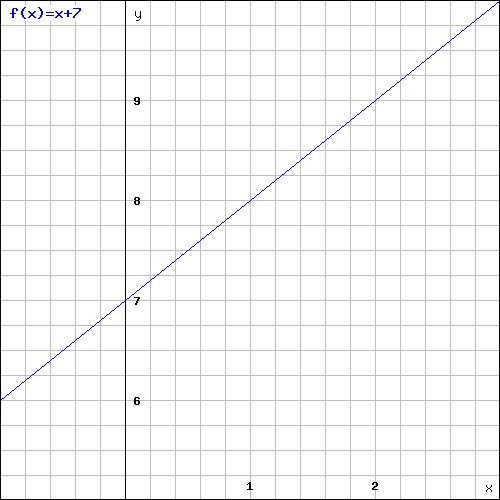
Functions And Linear Equations Algebra 2 How To Graph Functions And Linear Equations Mathplanet
Graph Of A Function Wikipedia
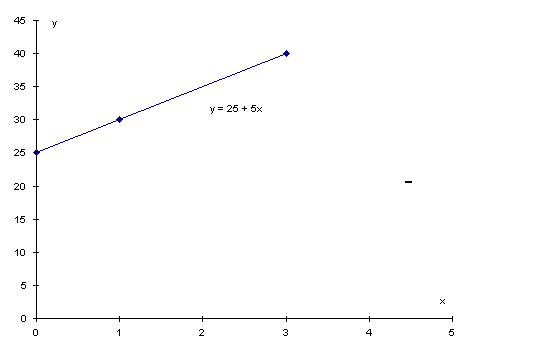
Linear Functions

Graphs Of F X F X F X And X 0 F T Dt For Example 29 5 Download Scientific Diagram
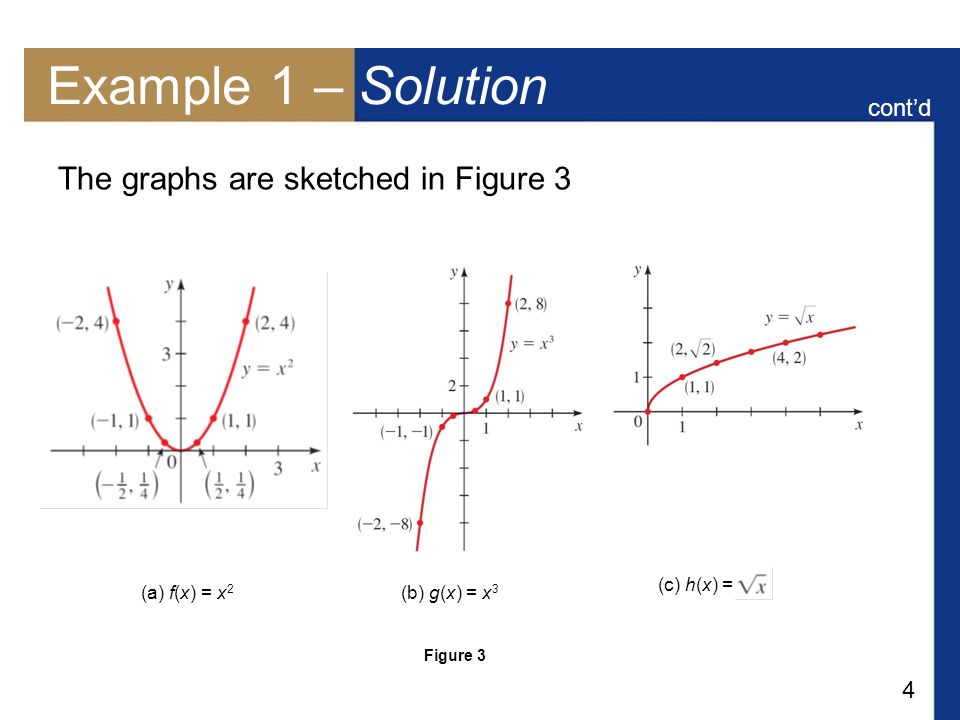
1 Graphing Functions By Plotting Points A Function F Of The Form F X Mx B Is Called A Linear Function Because Its Graph Is The Graph Of The Equation Ppt Download

Mhf4u1 8 1 Example P2

Graphing Functions With Excel
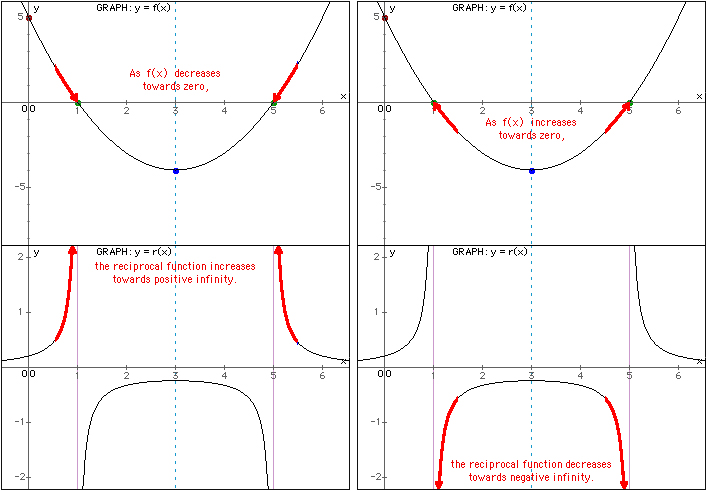
Asymptote

College Algebra Problems With Answers Sample 4 Graphs Of Functions

Finding Limits Using A Graph Mathbootcamps

Ap Calculus Review Estimating Derivatives From Graphs Magoosh Blog High School
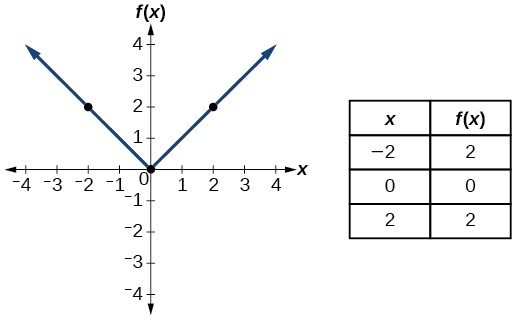
Identify Functions Using Graphs College Algebra
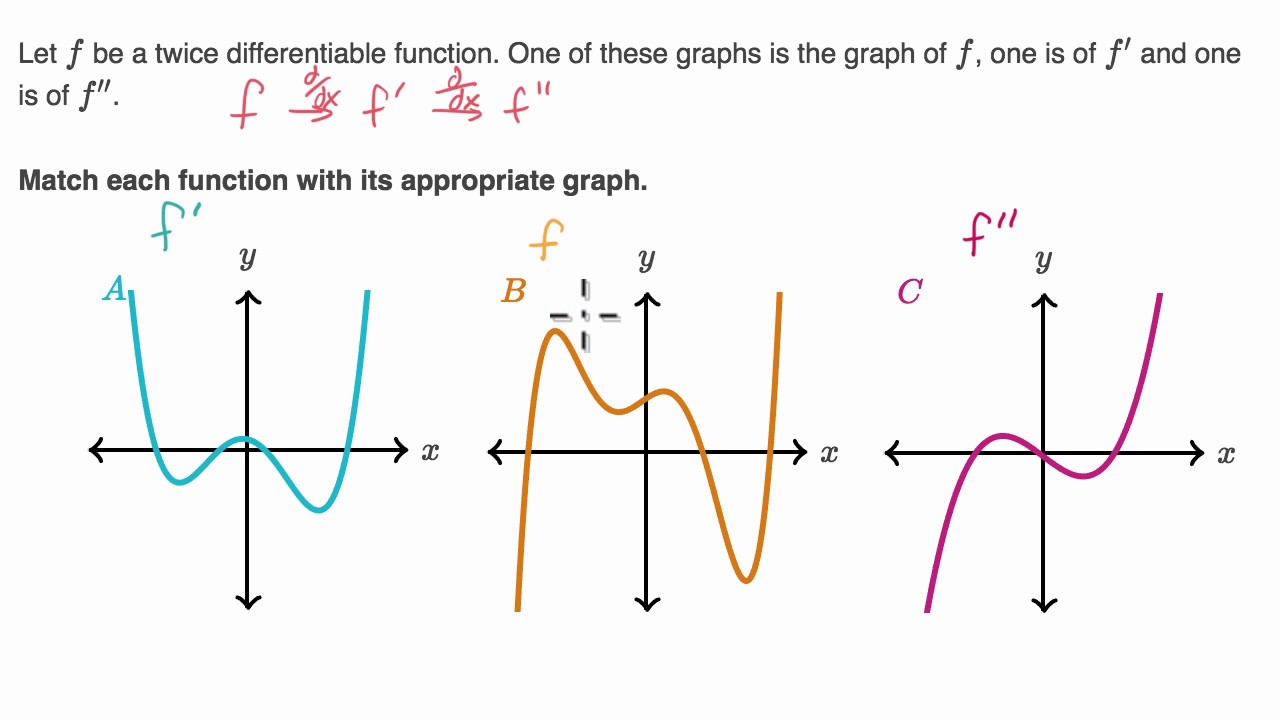
Identifying F F And F Based On Graphs Youtube

Vertical Tangents And Cusps

3 3 Domain And Range Mathematics Libretexts
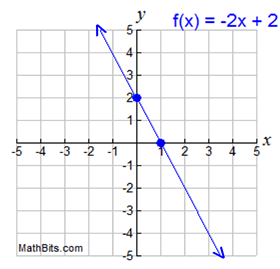
Features Of Function Graphs Mathbitsnotebook A1 Ccss Math
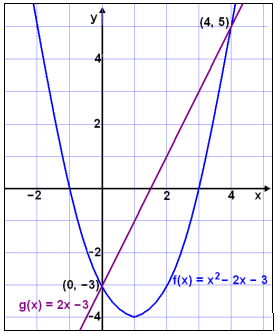
Math Scene Equations Iii Lesson 3 Quadratic Equations
Examples Friday Feb 21
Www Math Utah Edu Wortman 1050 Text If Pdf

Introduction To Limits In Calculus

Biomath Transformation Of Graphs
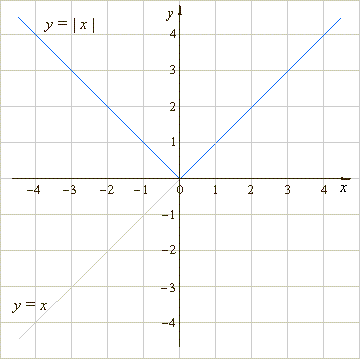
Quadratic Equations With Absolute Value

Finding The Limit Free Math Help
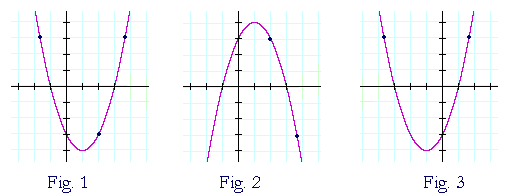
Reflections Of A Graph Topics In Precalculus
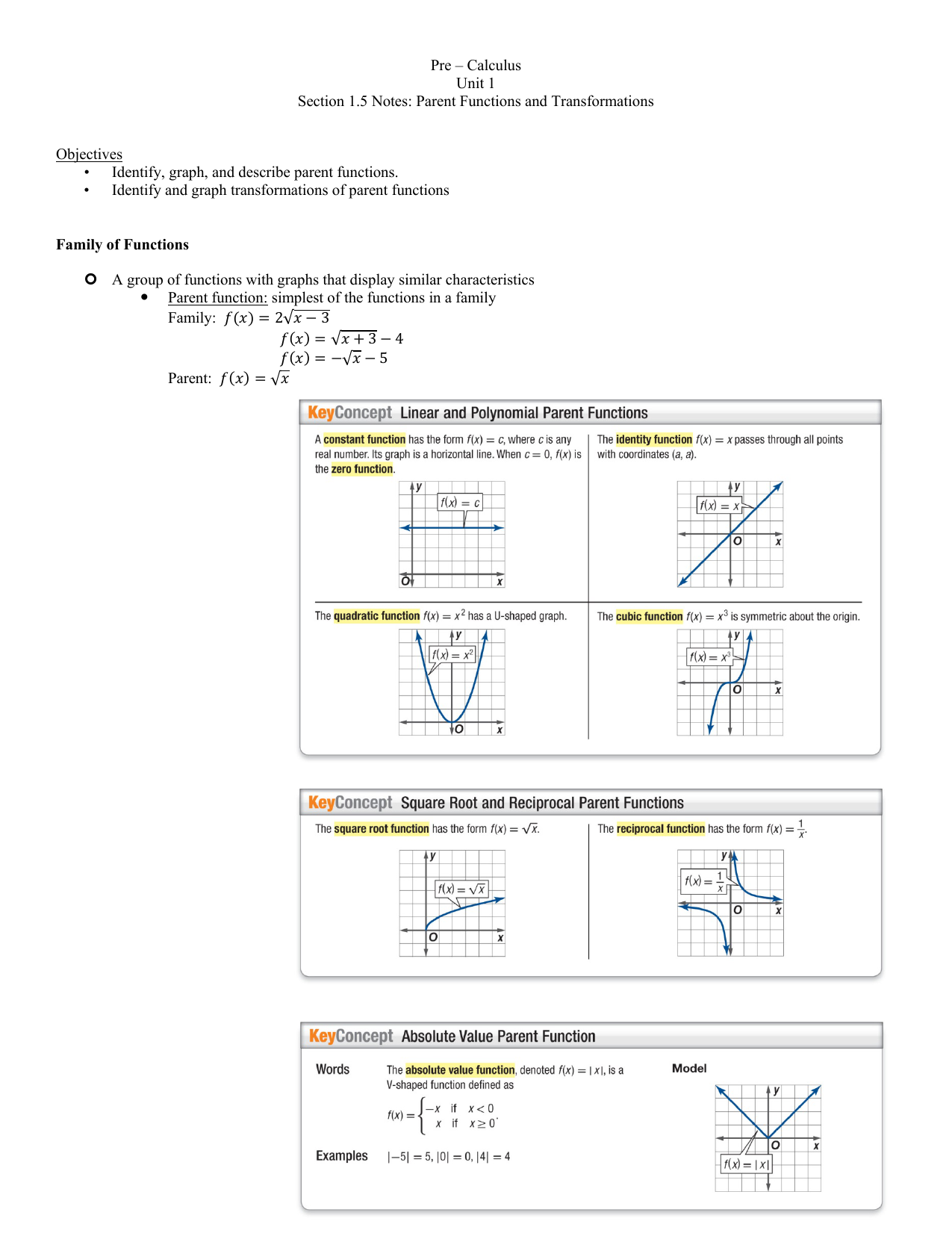
Parent Functions And Transformations Objectives Identify Graph
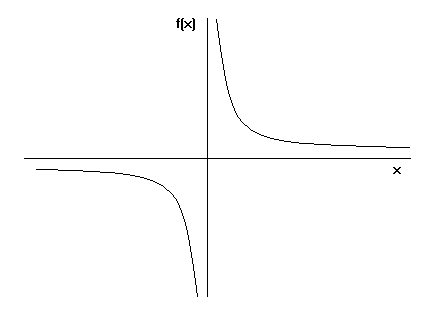
Functions Algebra Mathematics A Level Revision
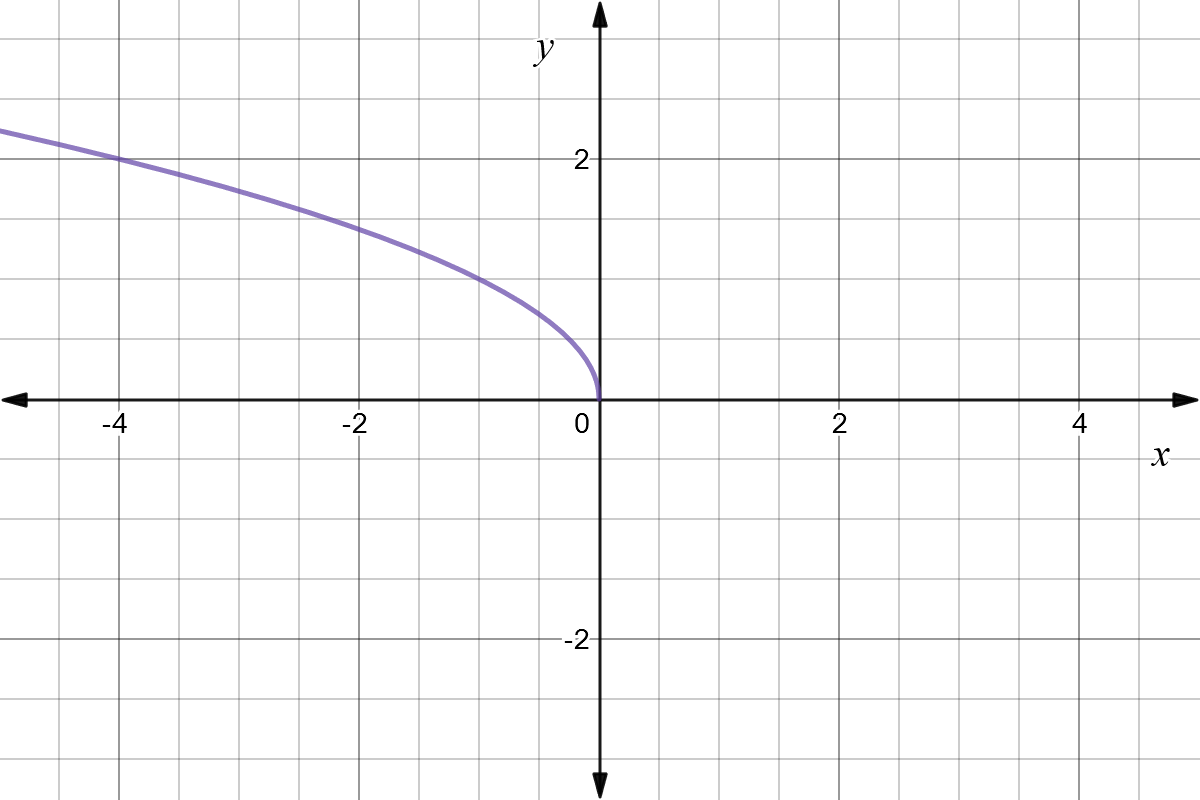
Reflect Function About Y Axis F X Expii

Function Notation And Evaluating Functions Mathbootcamps
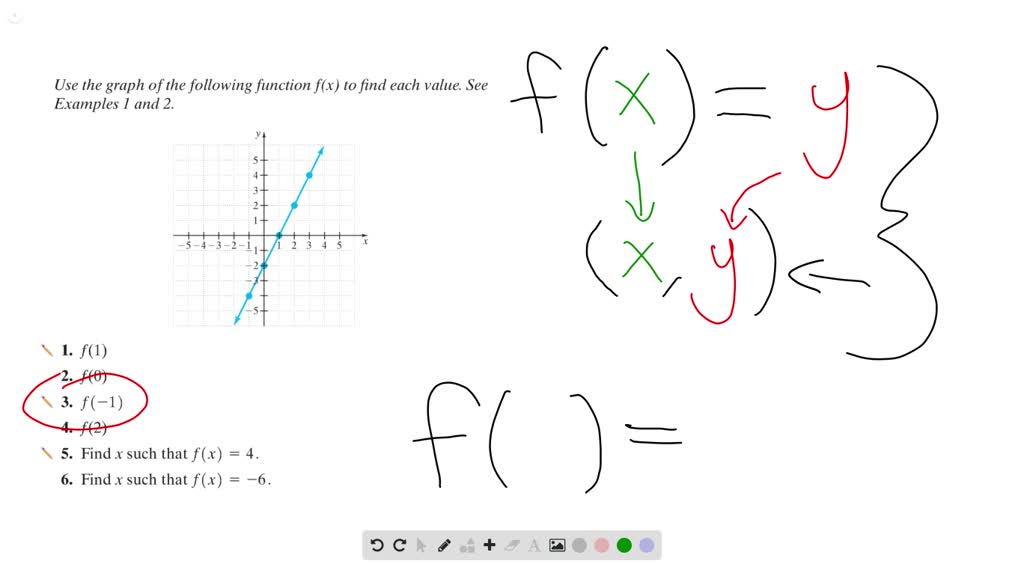
Use The Graph Of The Following Function F X To F

What Is A Power Function Definition Equations Graphs Examples Video Lesson Transcript Study Com
Matlab Plotting Tutorialspoint

Identify Functions Using Graphs College Algebra
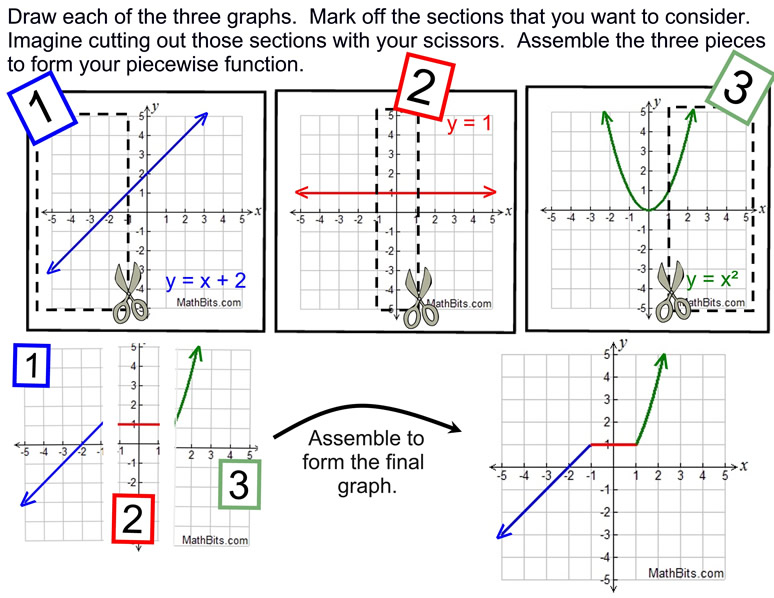
Piecewise Absolute Value And Step Functions Mathbitsnotebook A1 Ccss Math

Biomath Transformation Of Graphs
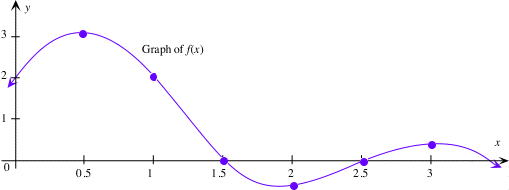
Graph Of The Derivative

Graph Of The Derivative

Piecewise Functions Examples
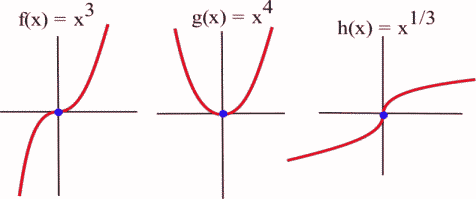
Business Calculus
Discontinuous Functions

Precalculus Shifting Reflecting And Stretching Graphs Flashcards Quizlet
Reflecting Functions Examples Video Khan Academy
Functions Inverse And Composite Functions
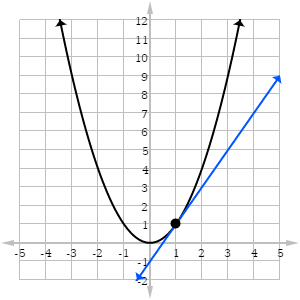
Ap Calculus Review Estimating Derivatives From Graphs Magoosh Blog High School

Answered 377 3 5 Rational Functions Graphs Bartleby

Graphs Of Functions
Web Ma Utexas Edu Users Jdanciger Fall11 Hw2 Soln Pdf
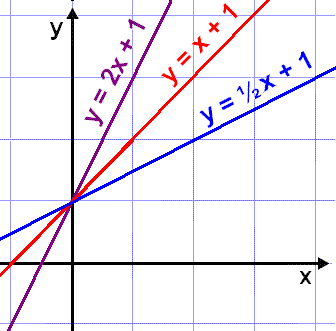
Math Scene Derivatives Lesson 2 Differentiating Polynomials

Graphs To Find Limits Ck 12 Foundation
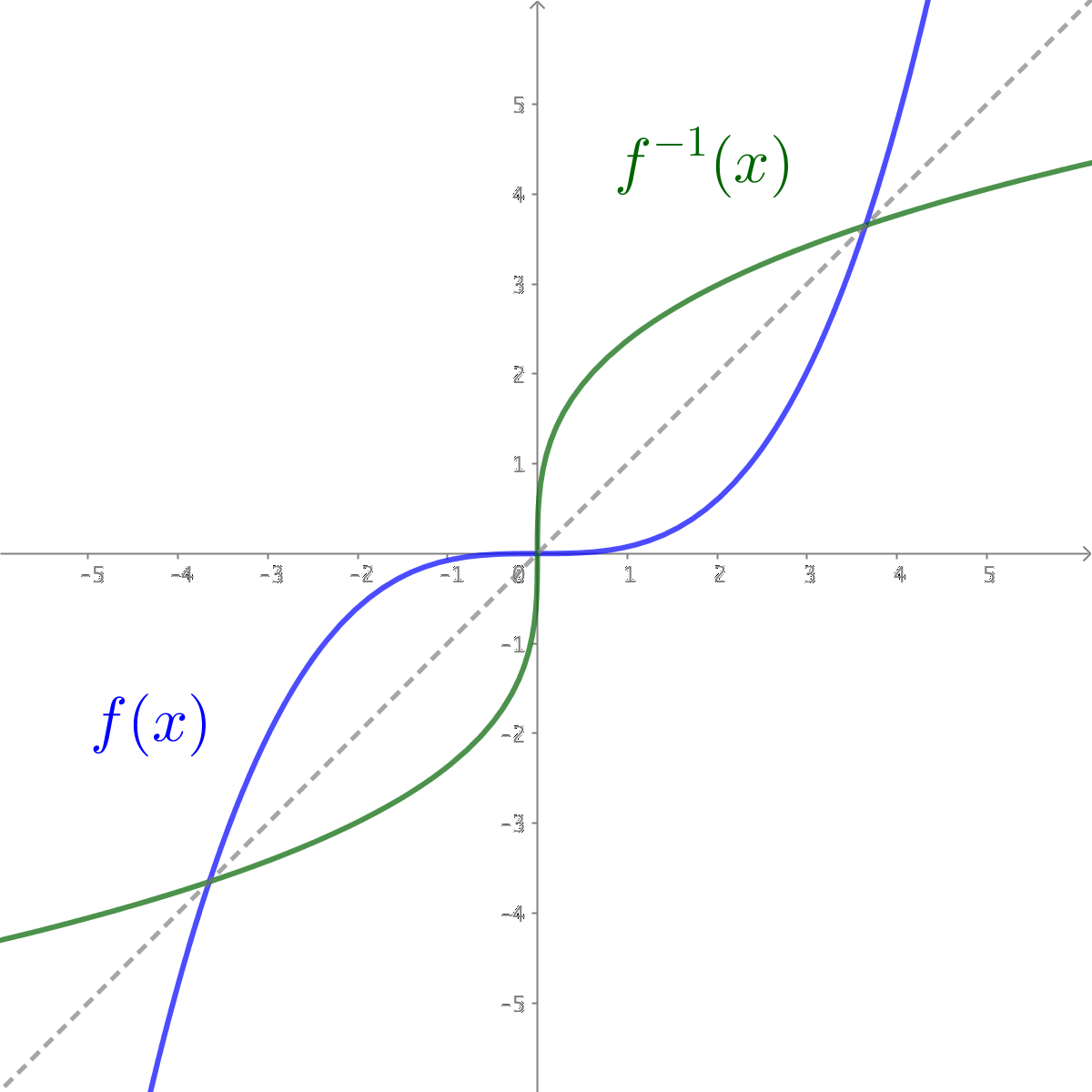
Functions Inverse And Composite Functions

Plot Expression Or Function Matlab Fplot
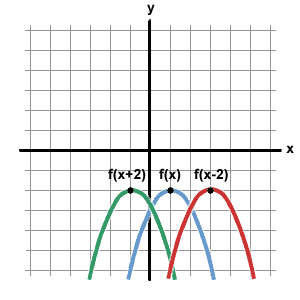
Operations On Functions Translations Sparknotes
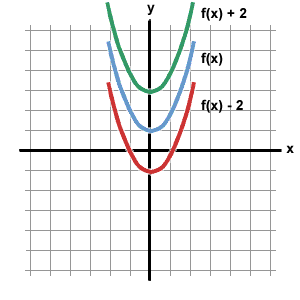
Operations On Functions Translations Sparknotes
Web Ma Utexas Edu Users Jdanciger Fall11 Hw2 Soln Pdf



